Dangers of the SCF-level DBOC: NO and NO2 as examples
James H. Thorpe, John F. Stanton
The Quantum Theory Project, Department of Chemistry, The University of Florida, Gainvesville, 32611
Email: james.thorpe@chem.ufl.edu
The diagonal Born-Oppenheimer correction (DBOC) [1, 2], which accounts for the finite mass of the nucleus, plays a small but vital role in high-accuracy model chemistries such as HEAT [3–5], W4 [6], and others. The considerable complexity and expense of obtaining this correction with a correlated theory, such as coupled cluster [7], has encouraged most authors to evaluate the DBOC with Hartree-Fock, where it is comparatively easily obtained from the CPHF solutions [8] as per Handy et al. [9]. However, as has been previously demonstrated for force constants [10, 11], these solutions are a poor treatment of what may be considered the ‘SCF excited states’, and CPHF poles, where the CPHF solutions approach a singularity, are frequent troublemakers in quantum chemical calculations of radicals.
That the seemingly innocuous SCF-level DBOC, which depends on the CPHF solutions, is susceptible to the same problems as force constants is apparently not fully appreciated. Here, the NO and NO2 radicals are used as case studies of such pathologies in the DBOC. The NO radical serves as an example of artificial fluctuations of the DBOC as a consequence of UHF instabilities, where better behavior is obtained at the CCSD and CCSDT levels of theory. The NO2 radical demonstrates a true divergence of the DBOC at the crossing point of the 2A1 and 2B2 electronic configurations, where the adiabatic representation of molecules breaks down and model chemistries built within the Born-Oppenheimer framework should be treated with great care. Using these two examples, diagnostic tools for potential DBOC breakdowns will be presented.
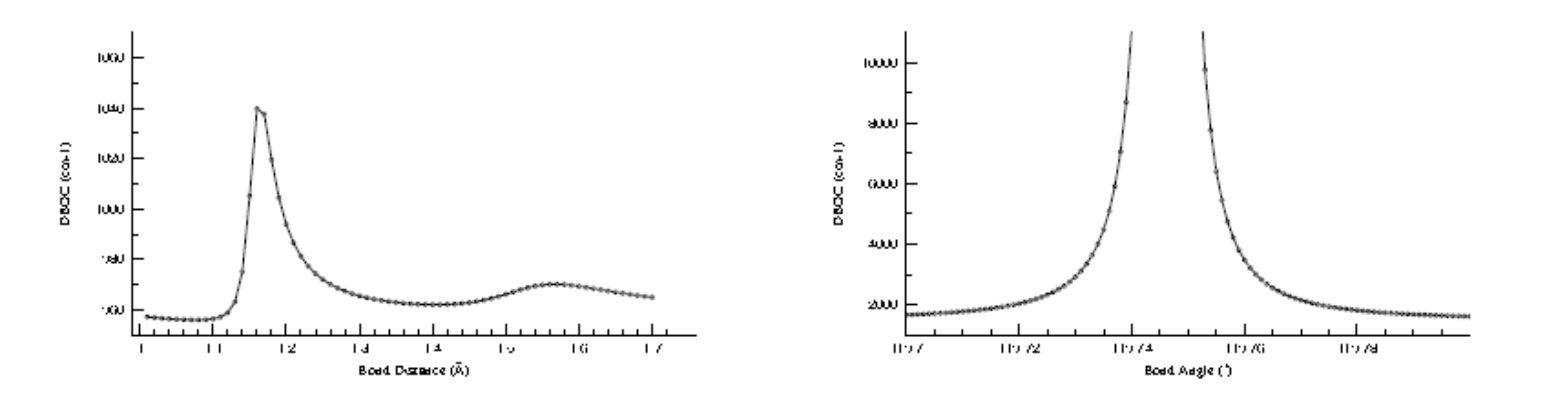
FIG. 1: Values of the DBOC (cm−1 ) as a function of bond length (Å) for NO (left) and bond angle (°) for NO2 (right).
[1] M. Born and K. Haung, Dynamical Theory of Crystal Lattices (Oxford University Press, New York, 1956).[2] H. Sellers and P. Pulay, Chemical Physics Letters 103, 463 (1984).
[3] A. Tajti, P. G. Szalay, A. G. Császár, M. Kállay, J. Gauss, E. F. Valeev, B. A. Flowers, J. Vázquez, and J. F. Stanton, The Journal of Chemical Physics 121, 11599 (2004).
[4] Y. J. Bomble, J. Vázquez, M. Kállay, C. Michauk, P. G. Szalay, A. G. Császár, J. Gauss, and J. F. Stanton, The Journal of Chemical Physics 125, 064108 (2006).
[5] M. E. Harding, J. Vázquez, B. Ruscic, A. K. Wilson, J. Gauss, and J. F. Stanton, The Journal of Chemical Physics 128, 114111 (2008).
[6] A. Karton, E. Rabinovich, J. M. L. Martin, and B. Ruscic, The Journal of Chemical Physics 125, 144108 (2006).
[7] J. Gauss, A. Tajti, M. Kállay, J. F. Stanton, and P. G. Szalay, The Journal of Chemical Physics 125, 144111 (2006).
[8] J. Gerratt and I. M. Mills, The Journal of Chemical Physics 49, 1719 (1968).
[9] N. C. Handy, Y. Yamaguchi, and H. F. Schaefer, The Journal of Chemical Physics 84, 4481 (1986).
[10] T. D. Crawford, J. F. Stanton, W. D. Allen, and H. F. Schaefer, The Journal of Chemical Physics 107, 10626 (1997).
[11] J. F. Stanton, The Journal of Chemical Physics 115, 10382 (2001).